
Forest Measurement and Modelling.
 |
Tree volume Forest Measurement and Modelling. |
     |
|
Stem volume is function of a tree's height, basal area, shape, and depending on definition, bark thickness. It is therefore one of the most difficult parameters to measure, because an error in the measurement or assumptions for any one of the above factors will propagate to the volume estimate. Volume is often measured for specific purposes, and the measurement and interpretation of the volume estimate will depend on the units of measurement, standards of use, and other specifications. For example:
Thus, the type of volume use measured must be reported for reliable interpretation. For example, the net merchantable volume of sawlogs in a tree will be significantly different to the gross biological volume. Calculations of merchantable volume may also be based on true cubic volume or product oriented volume. Product oriented volume is the volume of a nominal product that could be cut from the log or stem under specified conditions and assumptions. Direct and indirect methods for estimating volume are available. The direct methods tend to divide the stem into theoretical or actual sections and measure the volume of these sections:
The indirect methods include:
|
|
| Fluid displacement |
The fluid displacement method, also called xylometry, accurately measures gross biological volume. Essentially, the tree stem is cut into manageable sections and immersed in a bath. The amount of water displaced equals the volume of the section. This method does not require any assumptions to be made about tree shape and thus has no theoretical bias. However, xylometry is expensive and rarely used outside research. |
| Graphical method |
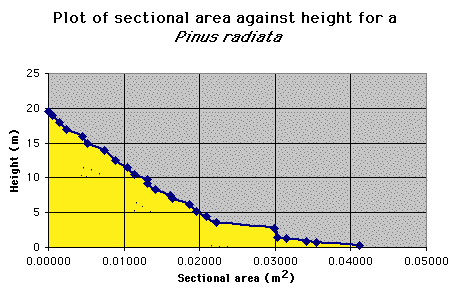 Measurements of sectional area (or diameter) are made every 1 - 2 m up the tree bole. When the sectional area (m^2) is plotted against height (m), the area under the curve is equivalent to the volume (m^3) of the bole.
Measurements of sectional area (or diameter) are made every 1 - 2 m up the tree bole. When the sectional area (m^2) is plotted against height (m), the area under the curve is equivalent to the volume (m^3) of the bole.Location of the measurements may be either objective (e.g. every 1 m regardless of local bole abnormalities) or subjective (e.g. avoid difficult to measure and abnormal positions on the bole). The objective selection of points would result in an unbiased estimate of biological volume, while the subjective points would underestimate biological volume. An estimate of bole volume from the graphical method is often used as the standard against which other estimates of volume are evaluated. However, the large number of measurements required makes this an expensive method. |
Standard sectional method
|
The main stem, up to merchantable height, is theoretically divided into a number of (mostly) standard length sections. The standard length is normally 3 m (10 foot). The exception to the standard section is the odd log - a section less than the standard length that fits between the last standard section and the merchantable height. These sections are assumed to be second degree paraboloids in shape. The bole from the merchantable height to the tip is assumed to be conoid in shape. Huber's formula is used to calculate the volume of the standard sections and the odd log:  where: v denotes volume (m^3), l denotes length (m), s(h) denotes sectional area (cm^2) half way along the log, and d(h) denotes diameter (cm) halfway along the log. A common error with this method is to mismeasure the odd log. The odd log begins at the end of the previous standard section (i.e. not at the point where that section was measured for diameter). The diameter measurement for the odd log must be midway between the end of the last standard section and the merchantable height. The volume of the conoid at the tip is calculated as: 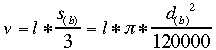 where: s(b) and d(b) denotes sectional area (cm^2) and diameter (cm) respectively at the base of the conoid. The merchantable volume of the bole is estimated by adding the standard sections and odd log volumes. The total volume is determined by adding the tip volume. Huber's formula is based on the assumption that the sections are second degree paraboloids. However, this may not be appropriate for the butt or base log - which is often neiloid. Huber's formula will underestimate the volume of a neiloid. However this underestimate will be small if the difference in diameter between the bottom and the top of the section is small (i.e. small rate of taper or small section length). Thus, sections smaller than 3 m may be necessary to avoid bias. Error in the standard sectional estimate of volume may also be introduced where the tip is not like a conoid. However the volume in the tip is relatively small, so this error is likely to be unimportant. A standard sectional method that used 1.5 m sections would provide estimates that were very close to those produced by the graphical method. |
|
Taper line method Gray's taper line 
|
During the 1940's, H. R. Gray hypothesised that the central section of a tree bole approximated a second degree paraboloid with a constant rate of taper. Thus, a few measurements of height and sectional area could be used to approximate the detailed and expensive stem profile derived by the graphical method for this section of the bole. If the butt of the tree were also assumed to be a second degree paraboloid, but with a different rate of taper, then an additional two or three measurements could approximate the shape of this section too. The tip of the tree could be assumed to be a final paraboloid which meets the central section at the point where the diameter is 10 cm (0.00785 m^2 sectional area). These assumptions allowed Gray to approximate the complete stem profile with only six or seven measurements. The measurements were also concentrated in the area below half the height of the tree (three or four measurements between breast height and half height were satisfactory for determining the straight line of constant taper for the main section). Volume was estimated as the area under the curve of sectional area plotted against height. Gray's Taper Line method is described here as an example of using well-established relationships to reduce the amount of measurement required for a given outcome. The method is not currently used. |
| Tree volume tables |
A tree volume table is a statement of the expected volume of a tree of nominated dimensions in a particular stand or population. The number of measurements or dimensions determines the number of entry points or ways into the table. Thus:
Before the general availability of computers, volume tables were compiled by summarising the volume of trees in dbh and height classes. Volume equations are developed now. The predictions from these volume equations are presented in tabular form for ease of use when field computers are unavailable. |
| Tree volume equations |
Like tree volume tables, volume equations are a statement of the expected volume of a tree of nominated dimensions in a particular stand or population. Volume equations are a very common approach to estimating volume of standing trees. The input variables to the equations can also include diameter (at breast height and other heights), height, taper, and interaction terms. Some equation forms have been given specific names:
In the above equations, v usually denotes total volume or merchantable volume to a minimum diameter that is defined as the merchantable small-end. An equation will need to be refitted if the merchantable small-end changes. Some of these equations are only appropriate when the merchantable volume is close to the total volume (i.e. merchantable small-end is 10 cm or less). Other volume equations include the merchantable small-end (s) as an additional input variable to allow different merchantable volumes (Vs) to be predicted (d denotes diameter at breast height, h denotes height, bh denotes breast height, and a1-5 denote constants): Alternatively, the total volume (v) predicted by above equations can be reduced by a ratio correction to estimate merchantable volume (Vs) to a diameter limit (s). The ratio (R) is simply calculated as: R = Vs / v. R is often correlated with diameter at breast height:
Care must be exercised in fitting and using all the above volume equations. In particular, local and general biases must be checked before the equations are accepted for use in a new area. |
| Integrating taper equations |
Taper functions effectively predict the stem profile for a tree. From this information, the area under a sectional area against height curve can be determined - this area under the curve represents the volume of the bole. Where a taper function is continuous and able to be integrated, the volume of the bole can be determined by integration. Where this is not feasible, the equation can be used to predict the sectional area at 1 or 2 m intervals up the tree, and volume can be calculated as for the [graphical method]. A major advantage of using integrated taper equations instead of volume equations is that volumes to different diameter limitations can be estimated. Thus, the volume of sections or products defined by the large and small end diameter can be predicted by a taper equation. The dependent variable volume in a volume equation is determined a'priori and the volume equation fitted only predicts this type of volume. Rivers (1995) found that taper functions could introduce bias into the estimates of the volume of tree sections. This bias was significant, even though the taper equation was unbiased in its estimation of diameter. |
|
Variance reduction Importance sampling Centroid sampling 
|
Variance-reduction methods use the knowledge contained in taper functions to improve the precision and cost of estimating sample tree volume. Essentially, a taper model predicts tree shape and hence sectional area and cumulative volume at any height up the tree. A sample height on the tree is selected and the bole measured. The difference between the measured and predicted size at that height is used to correct the volume estimated from the original taper equation. Various methods differ in the way the sample height is selected and the way the original estimate is corrected.
|
|
[volume.htm] Revision: 9/1999 |